Distributed area of the load. Evenly distributed load

When solving practical problems, it is not always possible to consider that the force acting on the body is applied at one point. Often forces are applied on the whole body area (for example, snow load, wind, etc.). This load is called distributed. An evenly distributed load is characterized by an intensity q (Fig. 1.29). Intensity is the total load per unit length of the structure.

Decision. We use the same plan that was used to solve problems on a convergent system of forces. The object of equilibrium is the entire beam, the load on which is shown in the drawing. We drop the links - hinges A and B. The reaction of the fixed hinge A is decomposed into two components -

and

, and the reaction of the movable hinge B is directed perpendicular to the reference plane. Thus, a plane arbitrary system of forces acts on the beam, for which three equilibrium equations can be formed. We choose the coordinate axes and compose these equations. Equations of projections:

1. F kx = 0; R ax -Fcos (60) = 0;

2. F ky = 0; R ay + R B - Fcos (30) = 0;

(the pair does not enter into the projection equation, since the sum of the projections of the pair forces on any axis is zero).

The equation of moments is made with respect to point A, since two unknown forces intersect in it. When finding the moment of the pair with respect to point A, remember that the sum of the moments of the forces of the pair with respect to any point is equal to the moment of the pair, and the sign of the moment will be positive, since the pair tends to rotate the body counterclockwise. To find the moment of force 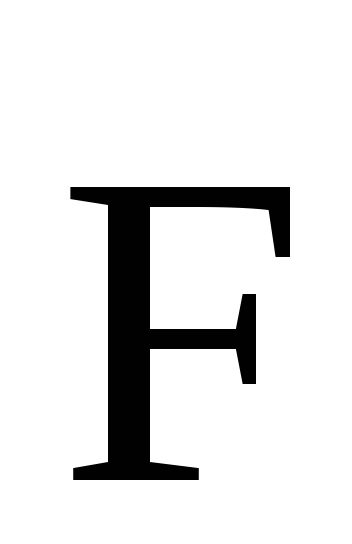it is convenient to decompose it into vertical and horizontal components:

F x = F cos (60), F y = F cos (30)

and use the Varignon theorem, and it should be taken into account that the moment of force with respect to the point A is equal to zero, since its line of action passes through this point. Then the moment equation takes the form:

3.

; R in. 3-F B cos (30) 2 + M = 0.

Solving this equation, we obtain:

From the equation (2) we find:

R ay = Fcos (30) - R B = 20.867 - 4 = -2.67 kN,

and from the equation (1) R ax = Fcos (60) = 20.5 = 1 kN.

Decision. We replace the uniformly distributed load of its resultant Q = 3q = 310 = 30 kN. It will be applied in the middle of the span, that is, at a distance AC = 1.5 m. We consider the equilibrium of the beam AB. We reject the connection - a rigid embedding, and instead we apply two components of the reaction R ax and R ay and the reactive moment M a. A flat arbitrary system of forces will operate on the beam, for which three equations of equilibrium can be made, from which the unknown unknowns can be found.

F kx = 0; R ax = 0;

F ku = 0; R ay - Q = 0; R ay = Q = 30 kN;

M a (F k) = 0; M a - 1,5Q = 0; M a = 1.5Q = 1.530 = 45 kNm.

Stress distribution in the case of a plane problem

This case corresponds to the stress state under wall foundations, retaining walls, embankments and other structures, the length of which considerably exceeds their transverse dimensions:

Where l - length of the basement; b - width of the foundation. The stress distribution under any part of the structure, separated by two parallel sections perpendicular to the axis of the structure, characterizes the stress state under the entire structure and does not depend on coordinates perpendicular to the direction of the loaded plane.

Let us consider the action of the running load in the form of a continuous series of concentrated forces R, each of which is per unit length. In this case, the stress components at any point M with coordinates R and b can be found by analogy with the spatial problem:

If the ratio of the geometric characteristics of the points under consideration z, y, bto present in the form of coefficients of influence K, then the formulas for the stresses can be written as follows:

Values of the coefficients of influence K z, K y, K yz tabulated depending on the relative coordinates z / b, y / b(Table II.3 of annex II).

An important property of the plane problem is that the stress components t and s y in the plane under consideration z0y do not depend on the coefficient of transverse expansion n 0, as in the case of the spatial problem.

The problem can also be solved for the case of a linear load, in any way distributed across a strip of width b. In this case, the elementary load dPconsidered as a concentrated force (Fig. 3.15).

Fig.3.15. Arbitrary distribution

load across the width of the strip b

If the load propagates from the point A(b = b 2) to the point B(b = b 1), then, summing the stresses from its individual elements, we obtain expressions for the stresses at any point of the array from the action of a continuous striped load.

For a uniformly distributed load, integrate the above expressions with P y = P = const. In this case, the main directions, i.e. directions in which the greatest and smallest normal stresses act, there will be directions located along the bisector of the "angles of visibility" and perpendicular to them (Fig. 3.16). The angle of visibility a is the angle formed by the straight lines connecting the point under consideration M with the edges of the strip load.

The values of the principal stresses are obtained from the expressions (3.27), setting b = 0 in them:

These formulas are often used in assessing the stress state (especially the limiting state) in the foundations of structures.

On the values of the principal stresses as semi-axes, it is possible to construct stress ellipses that clearly characterize the stressed state of the soil under a uniformly distributed load applied along the strip. The distribution (arrangement) of stress ellipses under the action of a local uniformly distributed load under the conditions of a plane problem is shown in Fig. 3.17.

Fig. 3.17. Stress ellipses under the action of a uniformly distributed load under the conditions of a plane problem

Using formulas (3.28), we can determine s z, s y and t yz at all points of the section perpendicular to the longitudinal axis of the load. If we connect points with the same values of each of these quantities, then we obtain lines of equal voltages. Figure 3.18 shows the lines of identical vertical stresses s z, called isobars, the horizontal stresses s y, called ruptures, and tangential stresses t zx, called shifts.

These curves were constructed by D.E. Polshin by methods of the theory of elasticity for a load uniformly distributed over a strip of width b, infinitely extending in a direction perpendicular to the drawing. Curves show that the effect of compressive stresses s z intensity 0.1 external load R affects a depth of about 6 b, whereas horizontal stresses s y and the tangents t propagate at the same intensity 0.1 R to a much smaller depth (1.5 - 2.0) b. Similar outlines will have curvilinear surfaces of equal stresses for the case of a spatial problem.

Fig.3.18. Lines of equal stresses in a linearly deformed array:

and for s z (isobars); b for s y (strut); in - for t(shear)

The influence of the width of the loaded strip affects the depth of stress propagation. For example, for a foundation 1 m wide, transmitting to the base a load intensity R, voltage 0,1 R will be at a depth of 6 m from the sole, and for a 2 m wide foundation, at the same load intensity, at a depth of 12 m (Fig. 3.19). If there are weaker soils in the underlying layers, this can significantly affect the deformation of the structure.

where a and b / are respectively the angles of visibility and slope of the line to the vertical (Fig. 3.21).

Fig. 3.21. Diagrams of the distribution of compressive stresses along the vertical sections of the soil massif under the action of a triangular load

Table II.4 of annex II shows the dependencies of the coefficient TO | | z depending on z/b and y/b (Fig. 3.21) for the calculation of s z by the formula:

s z = TO | | z × R.